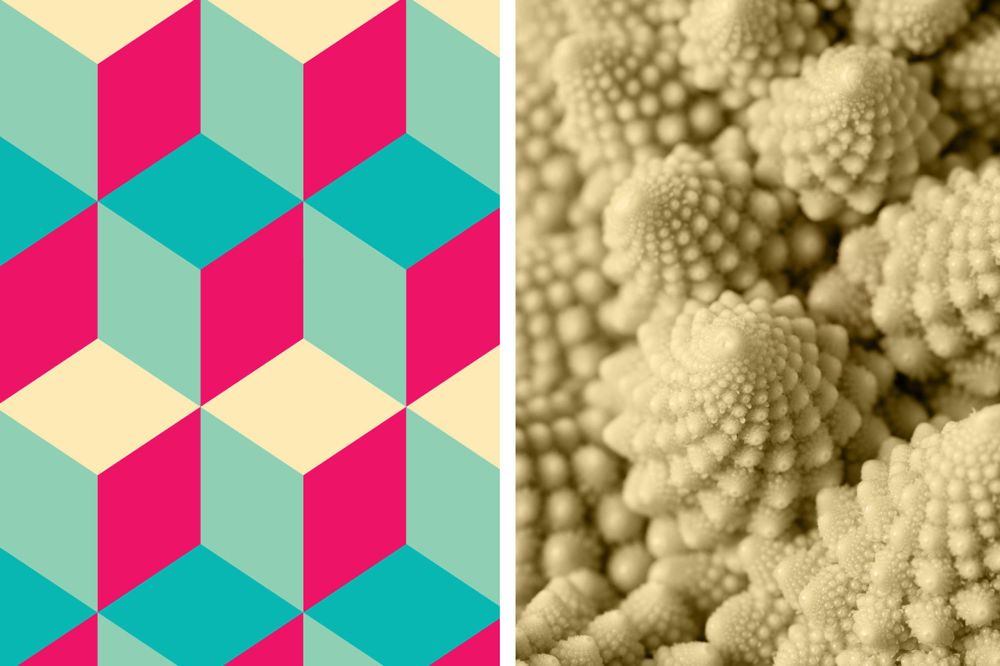
It is quite rare you’re ever going to have to use or come across fractals, whether it be art, geometry, mathematics, or otherwise. Tessellations, on the other hand, are much more common. A tessellation is the covering of a flat plane surface with one or more geometric shapes, in which there are no gaps. Things like a tile floor or a chessboard are an example of a tessellation pattern.
As tessellation is the much more common of the two, we’re going to be focusing our efforts on those for the majority of this article.
What are The Three Types of Tessellations?
There are three types of tessellations that you’re going to come across, and they are as follows:
Regular tessellations: Regular tessellations are tile coverings made up of only one shape. There are three types of regular tessellations, those being triangles, hexagons, and squares. These have interior angles which are divisors of 360.
Semi-regular tessellations: When two or three different polygonal shapes share a common vortex, it is called a semi-regular tessellation. There are nine different types of semi-regular tessellations that can be created by using various shapes at various lengths, such as combining triangles, hexagons, and squares.
Demi-Regular tessellations: These are the type that consists of two or three polygonal arrangements, of which there are 20.
What are Tessellations Used for?
Tessellation patterns can be seen in various areas of life, including in patterns and designs, hobbies, architecture, and also in the art of M. C. Escher.
You can commonly find examples of these in Islamic architecture, as no animals or humans are depicted on buildings with the belief that it might lead to idol worship.
- C. Escher used tessellation patterns extensively in his work, often to great effect. Many of his pieces featured animals using which he would divide the planes of the work. Escher was so into this style of artwork that he created his own new classification system for plane division.
What is a Tessellation Pattern?
A tessellation pattern, also called tiling, is when a number of different shapes fit together perfectly on a flat plane. There are three types that we have already discussed, and it is linked closely to hyperbolic geometry.
When a tessellation is created, we get a completely covered flat surface using various shapes. It is used in both math and art, although it is most commonly seen in the artistic world, with the likes of the previously mentioned M. C. Escher using it to accelerate his career.
Are Tessellations Math or Art?
The answer here is both. A tessellation is perhaps the perfect example of a link between the math world and the art world.
This link is highlighted by the fact that the three different types of tessellation have different mathematical equations, each of which on a different geometry plane including euclidean, spherical, and hyperbolic geometry, the latter of which was only first discovered in the 19th century.
It is a style that has been used in art throughout human history, dating back to Roman architecture, and even beyond that. The aforementioned Escher is the most recent example of a successful artist using tessellation and hyperbolic geometry in his work.
Escher produced his first tessellation in 1925. At the time it was a new and fresh entry into the art world and featured a series of interlocking lions, the first of a many number of works to use animals to divide the plane.
What About Fractals?
A fractal, on the other hand, is much more closely linked with math. A fractal is made by repeating a mathematical fraction repeatedly, so it cannot be created by hand. You’re not going to see any artists publishing fractal-based geometric artwork any time soon. The closest thing we’re going to get to that is one of the numerous Twitter accounts that post-AI-generated fractal artwork.
There are a number of computer programs dedicated to fractal artwork, so you can always go check them out if you’re interested in the designs for yourself.
When it comes to real-life applications and examples of fractals, you’re much more likely to find them in nature than you are in anything man-made. You can see fractal designs in a number of different types of plants and wildlife. Certain types of trees, broccoli, and certain cactuses all fit under the umbrella here.
That’s not to say that there are no practical uses for fractals, though, as it can be used to analyze seismic patterns and to model soil erosion.
Wrapping Up
To boil it all down, the primary contrast between them is that a tessellation is much more closely linked to the artistic world, whereas a fractal is far more mathematic in nature.
You are going to see samples of tessellation artwork far more in man-made objects, like architecture, tile flooring, designs on furniture, and so on.
Fractal artwork, on the other hand, is more likely to be found in nature, the natural world, and in computer programs.
Services
